Every once in a while, we hear something that is so out of the ordinary, so senseless and so frustrating that we feel an obligation to share it with others.
That was obviously the case when this image was posted on Reddit.
It was a mathematical question that appears to be on an elementary school quiz and the title was “The American Education System.”
Before we go any further, I should warn you that this particular math problem is going to blow your mind.
It’s not so much that it is difficult to figure out, it’s the fact that the educator could not step outside of their literal mind for two seconds to realize that the student was right.
This Is the Picture:

The question reads:
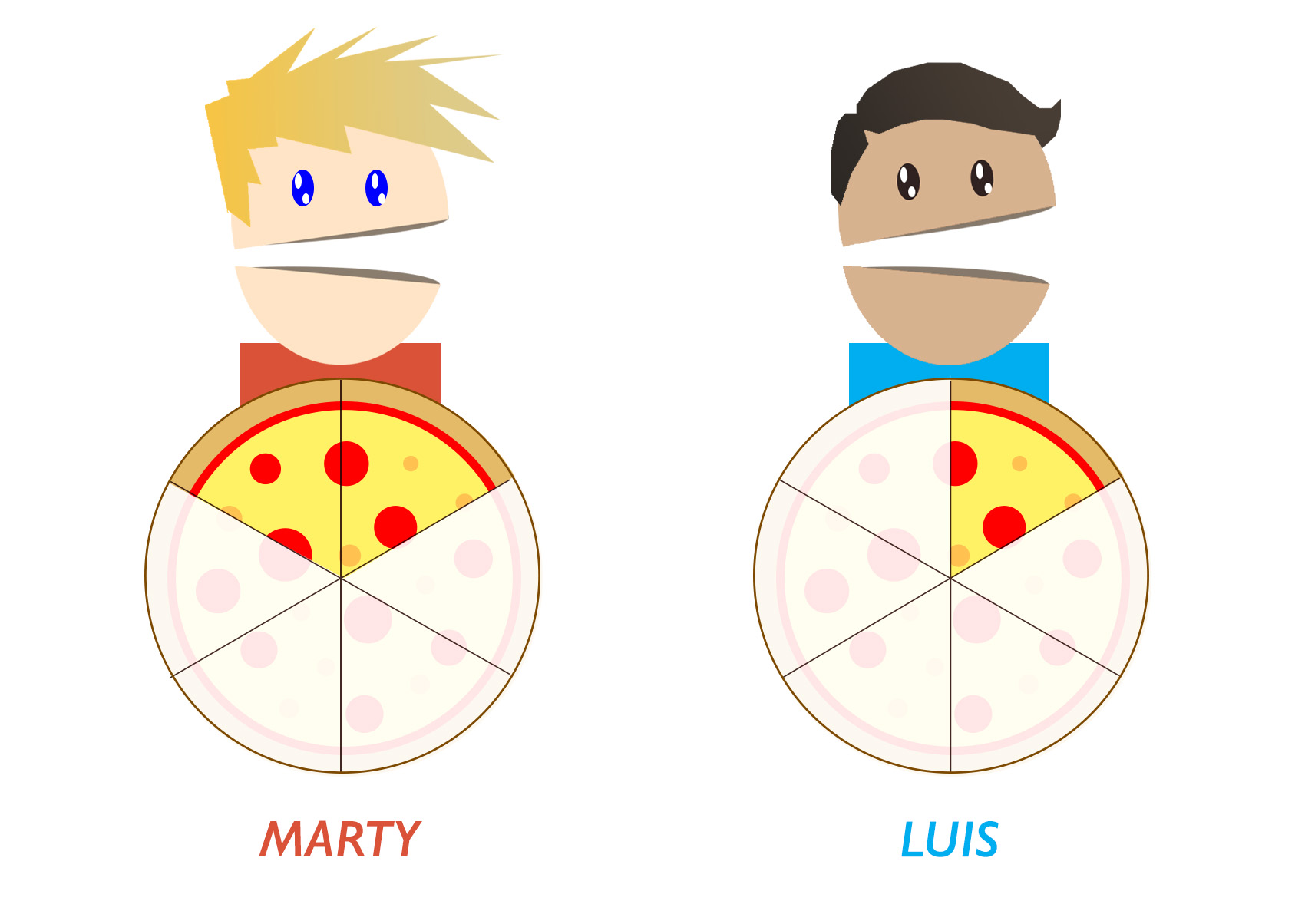
Reasonableness: Marty ate 4/6 of his pizza and Luis ate 5/6 of his pizza. Marty ate more pizza than Luis. How is that possible?”
The student gave the following answer:
Marty’s pizza is bigger than Luis’s pizza.
BRILLIANT! But then the teacher marked it wrong and gave the following explanation:
That is not possible because 5/6 is greater than 4/6 so Luis ate more.
The problem is not math, the problem is English. Both the question and the response are worded quite poorly.
This is why the question and the teacher’s answer are both horrible. This is also why the student was right.
A fraction is a unit of measurement, but a pizza is something completely different. There is no uniform size for pizza, it’s not an absolute unit.
The question does not state that the pizzas were the same size. The only information given to the student is about the fractions of pizzas that were eaten. As it is worded, it would require the possibility that the pizzas were different sizes.
This is what the question ACTUALLY says:
Marty has a pizza of unknown size that he ate 4/6 of.
Luis has a pizza of unknown size that he ate 5/6 of.
Marty ate more pizza than Luis.
Then the question:
How is that possible?
If the statements provided in the question are true and the facts are to be taken at face value, there is only one way that Marty could have eaten more pizza than Luis.
Quite simply, Marty had to start with a bigger pizza. If there was more pizza to start with, even a smaller fraction could add up to more volume.
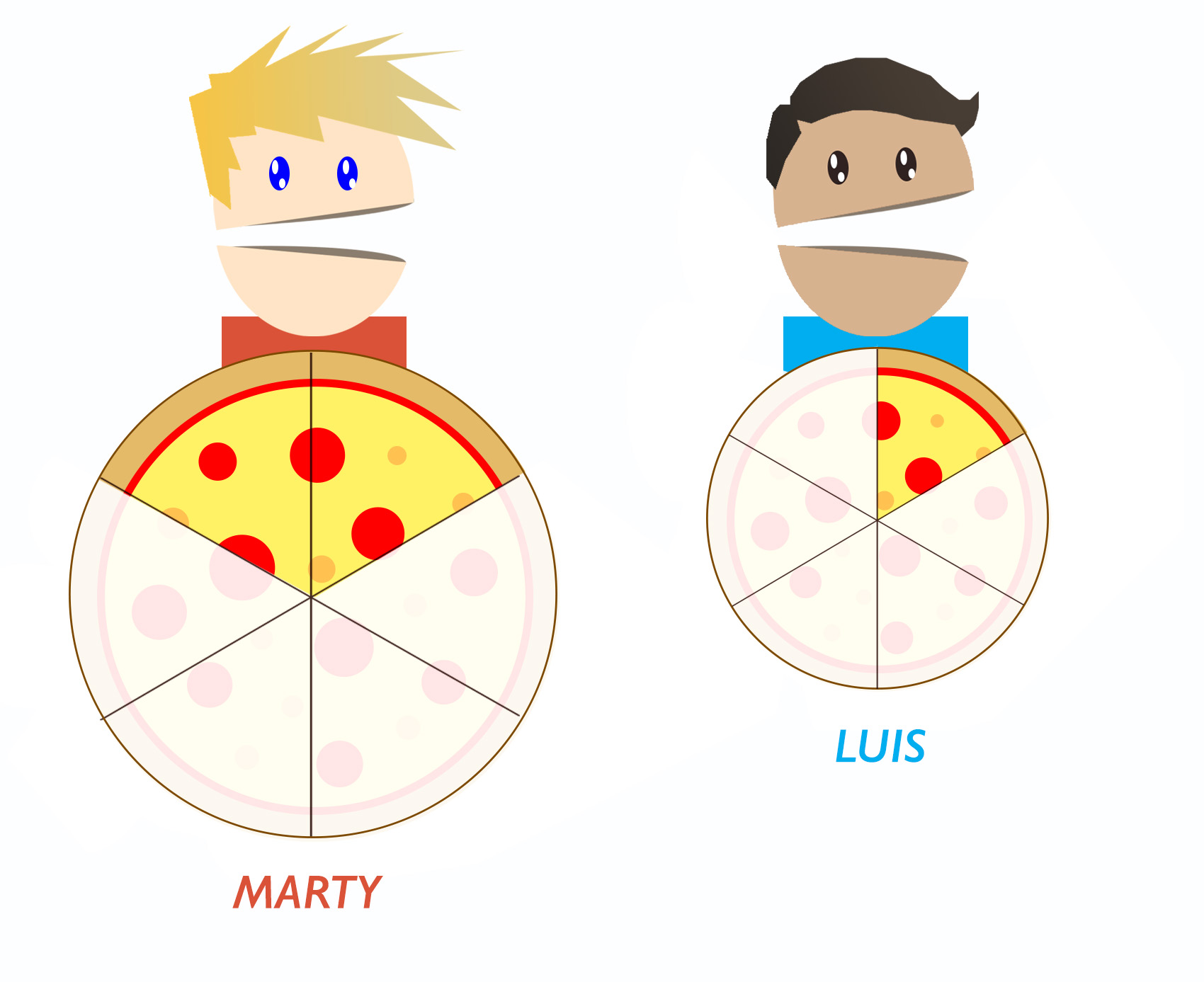
The only way that the teacher’s answer (“that is not possible because 5/6 is greater than 4/6 so Luis ate more”) could be correct is if the second statement (“Marty ate more pizza than Luis”) is false.
Admittedly, there could be additional answers, such as bringing a third pizza into the mix. But the evidence that was provided in the mathematical question means that the only possible answer is that Marty had a bigger pizza.
There is a counterargument, as is often the case. It involves the reason why the student is being tested, the concept of reasonableness.
The issue in this regard is that the student ignored the assumption that the pizzas are the same size and gave an unreasonable answer.
If that is the case, it doesn’t address the fact that the student’s answer had to hinge on one fact, that Marty ate more pizza than Luis. Otherwise, the question would be a lie.
Would it be “reasonable” to assume that the question was based on a lie and therefore should be ignored? I doubt it.
If the question had stated that both pizzas were the same size and then asked if it was possible, the answer would’ve been obvious. But as it stands, it seems as if the student gave a reasonable and correct answer to the question.
What is your opinion?
Be sure to share this on Facebook, along with your opinion.
Via: aplus

